The equation solver on the TI-84 Plus CE graphing calculator (and any other TI-84 plus graphing calculator, for that matter) is a great tool for quickly solving almost any equation you can think of.
The “Numeric Solver”, as it is called, is capable of solving any equation with a single unknown variable for all real solutions. The steps are simple. so let’s get started!
Solving Basic Equations
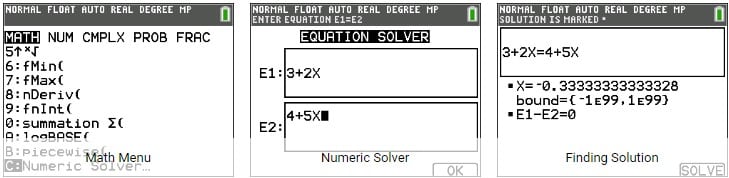
- Access the Numeric Solver by pressing the [Math] button, and then pressing the up arrow key to select the last option. Press enter to open it.
- You should now see the equation solver screen. The two boxes that are displayed represent the two sides of the equation.
- Type the left side of the equation into the E1 box. For example, if the equation was 3+2x=4+5x, you would type “3+2x” into E1. You can type “X” by pressing the [X,T,θ,n] key above the [Apps] button.
- Type the right side of the equation into the E2 box (you can switch between the boxes by using the arrow keys). For example, if the equation was 3+2x=4+5x, you would type “4+5x” into E2.
- Now that you have filled in both sides of the equation, press the [Graph] button at the top of your calculator to continue.
- You will now see “X=0” or x equals some other number. To calculate the actual value of X, press the [Graph] button once more.
- Congratulations! If everything went well, your equation should now be solved.
Solving Equations with Multiple Solutions
As you may have noticed, this program will not automatically provide you with all of the solutions to an equation: it will only give you the answer that is closest to the guess, the number that is typed in before you press [Graph] to actually solve the equation.
So, to find all of the solutions, just keep plugging in different guess values until you find all of them. The video shown at the top of this article has a good demonstration of how to do this.
Limitations of this Program
While this equation solver is definitely a useful tool, it does have some limitations. Here is a list of things that this solver cannot do:
- Equations with multiple unknown variables will not be solved correctly.
- Equations with infinite solutions will come up as having an answer equal to the guess value that is typed in.
- This program will often come up with solutions that are not 100% exact. For example, it might say the answer to “0=x-3” is 2.9999999789, when it is actually 3.